In this posting, David French makes excursion outside his field: patent law, to speculate on possible aspects relating to the physics and mechanism of the cold fusion phenomena.
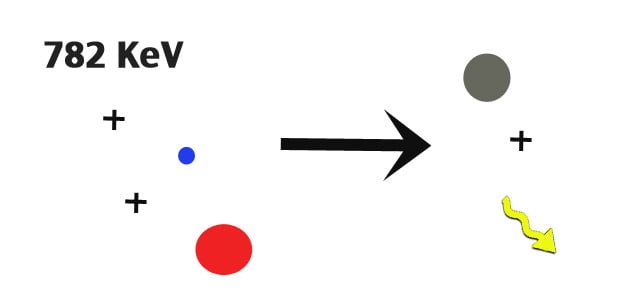
One of the theories to explain the ColdFusion excess energy effect is based on the premise that a proton can capture an electron, become a neutron, and then all sorts of magical things can happen. However, a neutron is heavier than the combined weight of a proton and an electron. The relative masses are:
Neutron = 1
Proton = 0.99862349
Electron = 0.00054386734
When you do the addition and subtraction it works out that a neutron is heavier than the proton-electron combination by a mass-energy equivalent of about 780 kilo electron volts ( keV). This amount of mass-energy must be found to make a neutron out of a proton and an electron. Here are some thoughts on that point.

When an electron falls from infinity towards a proton it acquires 13.6 electron Volts of energy to reach the ground state “orbital” around the proton. I have always wondered why it does not go all the way. Apparently, its Debroglie wavelength has to fit” around the “orbit radius” for it to occupy a stable state.
Perhaps another explanation is that an electron can only arrive in an atom and occupy an orbital by dissipating its arrival energy in the form of a photon. All the light we see originates from electrons settling into an empty slot in the shell of permitted orbitals around nuclei. If atomic dynamics do not permit the emission of such a photon, then an electron cannot settle into a stable orbital but must move on.
But what if an electron acquired enough energy to crash through the base orbital and proceed onward into a proton? How much more energy could the electron acquire hurtling towards the nucleus of a hydrogen atom? I have a suspicion that this might be a very large value if the radius of a proton is small enough.
Let us start by an analogy. Here is the formula for gravitational potential energy for a small mass “m” coming in from infinity to arrive at a radial distance “r” from a large mass M:
E = – GmM/r
This formula has an extraordinary consequence: if a mass were to fall to a point source where “r” drops to zero the energy would be infinite! This does not happen in the Sun, or even in the case of a penny being dropped down a very deep hole in the Earth. This is because as you go below the surface of the Sun or Earth the mass above you starts to cancel the gravitational force below you. Newton showed that there is no gravitational force at the center of the Sun or the Earth. The formula stops working when you reach a surface.
Let us turn to the potential energy associated with an electrical field. By integrating the energy acquired as an electron falls in from infinity, the amount of energy that it acquires as it approaches a proton is given by the following formula:
E = kQq/r
where Q and “q” are the sizes of the respective charges and “k” is a constant.
It will be seen directly that this formula parallels the one for energy acquired through gravitational attraction.
Again we are presented with the possibility that “r” might go to zero. Why is this important?
Well, Widom & Larsen postulate that an electron can be captured by a proton in order to become a neutron. But this requires approximately 780 keV, the mass difference between a neutron and the total mass of a proton and electron.
(I note that it has been said in Wikipedia about electron capture: “A free proton cannot normally be changed to a free neutron by this process; the proton and neutron must be part of a larger nucleus.” No reference is given for this statement.)
This large energy gap which is based on the mass difference between a neutron and a combined proton and electron has always seemed to me to be a barrier to electron capture by a proton. Since an electron only acquires 13.6 V falling from infinity to its ground state, it has got to acquire a lot more energy to get up to 780 keV. On the other hand, when “r” gets small, this kind of energy could be acquired quite quickly if the formula for potential electrostatic energy does not break down.
The gravity we experience from the Sun is the accumulation of force from the distributed mass contained in a body having substantial dimensions. It is not a point source. (Maybe a black hole is a point source!) But a proton is very nearly a point source. What does this size say about the potential energy that could be associated with the electrical attraction that extends between a proton and electron? Now let me take you on a little excursion concerning Blacklight Power and Randell Mills.
Randell Mills has his theory that electrons can occupy orbitals that are below the normal base orbital for a hydrogen atom. Randell calls such a special hydrogen atom a “hydrino”. Perhaps he has part of the explanation. I have met with Randell back in 1980’s and here is what he explained to me.
Electrons cannot fall below the base level in the normal hydrogen atom because they cannot emit a photon on their own. For his hydrinos to form there has to be a resonant absorption of energy from a nearby atom in order to permit an electron to drop below the normal base state. When falling through energy levels into an atom from infinity, an electron emits a photon to dissipate its acquired energy.
Apparently, once an electron reaches the base orbital, it is no longer capable of emitting photons as a way of losing energy. But according to Randell if a nearby atom is able to eject an electron, acting as a “catalyst”, it may serve to allow a proximate electron that is in the base orbital of a proton to fall to a lower energy level, closer to the proton. The energy that is associated with the electron falling through the electric field towards the proton is released through the resonant absorption of that energy by the nearby “catalyst” atom which disposes of the energy by ejecting one of its electrons. Here is a description of his theory from the web:
“According to Dr. Mills, when a hydrogen atom collides with certain other atoms or ions, it can sometimes transfer a quantity of energy to the other atom, and shrink at the same time, becoming a Hydrino in the process. The atom that it collided with is called the “catalyst”, because it helps the Hydrino shrink. Once a Hydrino has formed, it can shrink even further through collisions with other catalyst atoms. Each collision potentially resulting in another shrinkage.”
From the same source:
“For those of you with a mathematical bent, the formula is ((2 x n) -1) x 13.598 eV, where “n” is the level number. (BTW the maximum level number is certainly no larger than 137, and may well be less than that, not least because when a Hydrino gets very small, it may undergo fusion reactions with other atoms.) Of course, the numbers can be added up. IOW if you start with a Hydrogen atom, and end up with e.g. a level 5 Hydrino, then you get a total of 41 + 68 + 95 + 122 = 326 eV. The total for any level can be calculated with the formula (n^2 -1) x 13.598 eV.”
[End of quotation]
Well, 137 x137 = 18769 electron Volts and (n^2 -1) x 13.598 eV gives 18769 – 1 × 13.598 = 255,207.264 eV.
This is a value which is well on its way to 780keV!! I do not know why the limit in the above formula is 137, but let us accept that for the moment. Using the formula for the potential energy that becomes available when two electrically charged bodies are brought into close proximity to each other, namely E = kQq/r , it may be that this requisite energy condition is within reach of some force or effect originating from within the proton. At that moment, the magical conversion into a neutron may occur.
Maybe having fallen to level 1/137 an electron is able to fall further into a proton, eventually contributing the additional energy that it acquires into a quark conversion that changes the proton into a neutron of higher mass, and then the electron simply disappears!
On the other hand, there may be some other principle or limitation that would forbid such an event. Still, it is interesting to muse on the consequences an energy formula that includes the remarkable factor: 1/r.
Persons wishing to make comments on this posting are invited to visit the Cold Fusion Now website where this article is posted.